What is logic?

Earlier I said that,

“You rest at the outset of an expanding logical world. Or of worlds of worlds of worlds over and again.”

Therein I have placed the core strains of this present search. Most obviously, we are caught between a world and worlds of worlds of worlds. The solitude of the One pit against the clamor of the Many reappears here, as it always has in human thought, the question of choosing either being our perennial friend and enemy. To choose today, if ever our choice, it was sought to clarify the fog around that thing which is called logical world. What space does it occupy, and wherein is it kept? In the head, on the page, or somewhere beyond our impoverished frame of perspective? And also—what are the logical world’s constituents? Who are its dwellers? I am asking really, or I think,

I have said as well that this world(s) is expanding. How so, and what of it? Are we to be so impressed if there are more things but have yet gained nothing? That is for you to answer. I will insist only that its multifariousness, its explosive expansion destroys as it creates, and we gain precious much.

Returning to the world alone, what relation does the logical world bear on the world, or vice versa? Does the world define the logical? Does the logical speak of the world?

Can there ever be an outset to the logical world? Are there bounds, and if so, where do they lie? Or else, are we to think of it as all-encompassing and forever boundless?

At the end of the day, in magnificent reversal, what of yourself? What is your relation to the logical? How do you see it, how do you use it, and how can it so impress upon you the way it does?

Monism & Pluralism

The One and the Many: what do I mean? Of what is there One or Many or? I mean only to ask the question

Could there be/one true logic/to rule them all?

Surely you understand the difference between a tyrant and many. But you must wonder at the curiosity that is ‘true logic’. If you were Frege or Russell, ‘true logic’ might have been some kind of redundant redundancy. One kind of curious curiosity. To Frege and Russell the logical at its barest, in all its unadorned nakedness, is a body of truths.¹The logical fundamentally structures and embodies itself around a particular class of truths, namely the laws of truths or even, the truths about truths.²So then logic cannot help if it is true. Truth is simply its reason for being.

But this is such an old conception of logic, and we have long left that frame.

do not mourn its obsolescence. everything goes that way in time. at least the frame still hangs on a wall somewhere and remains open for viewing like all venerated and displaced historical treasures.

But moving now to stand before the present, there are four things I should like to tell you.

Four things of consequence:

1. We are currently situated in that frame where logic’s most fundamental core is consequence.³
2. Consequence — that primordial, chaotic concept from which the logical world is forged and cools over.
3. So, we are currently situated in that frame where logic’s most fundamental core is that primordial, chaotic concept from which the logical world is forged and cools over.

This last thing cannot be seen with simple blackened words. Be assured that it is there as I am here. I am eluding you, of course, but this frame eludes only because a frame becomes indiscernible when you yourself sit inside its room. Let me put it another way. Churning 1 and 2, which alone do nothing, and then to mingle them—to disturb their natural nothingness—to form 3: this is the creation of consequence. It is an inventive act and purely constructive as it builds new facts upon the old; or at least, it reveals the old’s new faces.

Yet for all that it creates, we stand not amazed. For the drawing of consequence is to draw in midair. The process is unseen, though its product is seen, and there is little time to hold sacred forms we cannot even imagine. though little time has not kept some from trying. Conjoined with consequence’s invisibility is the fact of its inevitability. We are moved, pushed even, to find thought’s end, otherwise known as its conclusion, once we are on that road. Can you escape 3 were it the case 1 and 2? There is simply nowhere for you, this day or tomorrow.

Thus is the matter: so ordinary, so banal to us is consequence that we usually do not note its procession, and we leave its mark off the page.

But as I said, consequence is logic’s most fundamental core, and so it must mark consequence’s revered presence one way or another. Of course the logician cannot help doing so. She would have done this in many ways:

Here already is one thing you may learn. Logic seeks to make visible what was latent, ignored, and taken for granted. It seeks to clarify and uncover what was crouched and shrouded. No wonder the logician has few admirers though she helps many.

That their thoughts, or that they themselves, are bare in daylight?

But clarity is the very design of logic: to know what follows from what and how so,

(it leaves little room to hide, and this is undesirable to us, we people who like to hide, as i hide now within a bracket.)

Why have I taken these pains to speak to you of consequence?

BECAUSE

It is the core concept of the logical: yes, how many times am I to convey this to you?

Consequently, this is what we mean when we say “there is only one true logic,” or “there are many logics.”

I mean that “logical pluralism is a pluralism about consequence,” and logical monism is the contrary thesis.⁴

Now in words that are more mine:

Dread is to choose between two roads that appear always to promise something when, in fact, they promise nothing. Here, two lines are monuments to fragility and limitation, as well as the hope we hold on the way. Can you see now what else may two lines become? You may imagine a form, a word, or a world. And when we are extraordinarily lucky, two begets three, an offspring set of points to tread:

In a yellow wood,
the gap where two roads diverge,
there waits another question.
Do you follow me there?

On Following

“Thus,” “consequently,” “as such,” “therefore,” and “it follows that” are all phrases that denote roughly the same thing: consequence.

It seems that concept of consequence is definite and precise, just as definite and precise its token is underlined there. However, this seeming does not coincide with reality (or at least what I think is reality, but I reserve for myself the thought that reality is just what I think).

Because though the concept has a formal use, it first lived in the domain of our ordinary and everyday intuitions.⁵These intuitions were given to us just as they were given to our forebears and they their forebears; no one can know just how old they are.

Because its foray into the formal domain was pushed on by people&people are such that they often make arbitrary choices. And besides, these are people who sought to reconcile the content of the everyday concept with the formal, precise one.⁶

“The concept of following is not distinguished from other concepts of everyday language by a clearer content or more precisely delimited denotation, the way it is used is unstable, the task of capturing and reconciling all the murky, sometimes contradictory intuitions connected with that concept has to be acknowledged a priori as unrealizable, and one has to reconcile oneself in advance to the fact that every precise definition of the concept under consideration will to a greater or lesser degree bear the mark of arbitrariness.”⁷

There. I have used the weight of another’s word: am I to be more or less trusted? For if I am helped and am more trustworthy to you, then that means my own word is not enough and should not be trusted at all.  Against arbitrariness and murk, the bedrock safety of a theory may give you short peace.

A Theory of Following Logically

“We say that the sentence $X$ follows logically from the sentences of the class $\mathfrak{R}$ if and only if every model of the class $\mathfrak{R}$ is at the same time a model of the sentence $X$.”⁸

We call such a theory semantical, the heads to the tails of syntax on the coin of meaning.

1. HEADS: TAILS

   A) OBVERSE: REVERSE

   B) VERSO: RECTO

   C) CONTENT: FORM

   D) SEMANTICS: SYNTAX

Shall we flip our coin to make the choice?

ALL, and we proceed.

I mentioned ‘peace’? I am beside myself; it was a misstep. I did not mean it in sincerity even if peace were true. I could never give you such lightness of being or such heaviness of solace, so do not feel that you are my debtor. I give you my word, and this time, it is sincerely for you. That is, what I can give to you is what I express, and what I express—my meaning, my content and discontent—is held together by the bindings of form and ruled structure.⁹ If I have said something—something of meaning—it was carried to you across time and place in these wrappings and is your present BURDEN. No doubt, I am restlessly concerned that if I have said something, it is something much like nothing. It is a worry owing to our seat in time and place, WHICH IS NONE OF YOUR CONCERN. It will push against you, the weight of what I mean, and I pray you shall _sink there. Such is my cruelty. though surely it is only human need.

It is not formless: believe me, meaning has its body too.
(how else could it have form? how else could it have weight?)
It is just that some bodies make themselves more known than others. But meaning must have it, for without body, there would be only abyss. Hold yourself over it now, look down, and do not fall.

What if content and meaning is never without form and body? What if the gulf between them is actually one quiet, unidirectional continuous stream? This I think when staring into a little pond of reflection.
Then would evaporate our two-sided coin, SEMANTICS: SYNTAX.
Or maybe it sinks into our stream, SEMANTICS—SYNTAX.
But that is only my pond; I know not others.

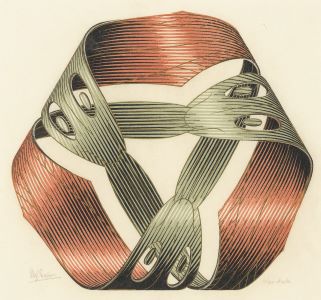
Möbius Strip I by M. C. Escher. The National Gallery of Art.

Do you hear me? Stand if you are unhurt.

Before that semantical theory of following logically, there was a syntactical theory.¹⁰ It was thought that one thing follows some others if it could be derived from those others using the rules.¹¹

BUT THE RULES DID NOT AND COULD NOT SUFFICE.

For, how could inference rules give us the means to derive “Such and such holds for all humankind,” from

“Such and such holds for the first person &

the person after the first &

the person after the person after the first &

then the person after that one &

... &

... &

... &

it holds for the last person, who has just now joined us in life and cries over his gain and loss.”¹²

Yet we feel that “Such and such holds for all humankind,” must follow were we able to tell it for every human who’s fortune is to inhabit this Earth.

Even after adding structural rules such as induction, whose power is to obtain “Such and such holds for all humankind,” THE RULES STILL DO NOT SUFFICE.

For, “if we abstract from certain theories with a very elementary structure, then always—no matter how we enrich the stock of rules of inference—we shall be able to construct sentences which follow in the everyday sense from the theorems of the deductive theory under consideration, but which cannot be proven in this theory on the basis of the accepted rules.”¹³ See here, a serendipitous meeting with the mirror: the INCOMPLETENESS of the syntactical theory follows from a certain kind of INCOMPLETENESS of our basic formal languages.¹⁴

Thus, we leave the syntactical theory (of) following behind us. Though the semantical theory does not fall behind in this way, and though it agrees with our old intuitions

& though for the concept of following logically it makes necessary and insufficient the property $F$ that deliberate substitution of non-logical constant terms for other constant terms in $X$ and in the sentences of class $\mathfrak{R}$ preserves $X$’s truth so long as every sentence in $\mathfrak{R}$ is true,

we arrive at the yellow wood, and our question is here.¹⁵

The virtue of the semantical theory rests in making $F$ necessary but insufficient. Yet like all virtues, here too lies vice; they, of course, sleep in the same bed and warm each other by their closeness.

The vice we currently face is that $F$ depends upon a division of all our language’s terms into the logical and non-logical. However, the possibility of division is precisely our question, AND THE ANSWER IS THAT THERE IS NO SOLID LINE BETWEEN THEM.¹⁶

Thus,
consequently,
as such,
therefore,
and it follows that

that primordial, chaotic concept from which the logical world is forged and cools over,

is not merely primordial and chaotic:

it also makes us look far beyond a yellow wood

where we discover that our fundamental question will never yield itself.

Now we may face with awe the fog that surrounds

and try with the means we have to approach

even if we should always remain at its fringes.

It is simply an astonishing inversion.

REMEMBER THAT IT WAS ONCE SOUGHT TO CLARIFY THE FOG THAT HANGS AROUND THE LOGICAL WORLD.

Though we have managed to utter some things, we have not said enough to dispel it completely. SO TODAY WE LET OURSELVES BE SUBSUMED BY IT.

Do not laugh. You think I am offering a false mountain mysticism.
I am not—you will never understand my aims.

I pity that you think to accept the surrounding fog is a humiliating defeat. I pity that you think our ending must be here.

And I pity most of all that you would rather stand helpless in trepidation when faced by expanding daze. Why have you thought that fog means there is nowhere to go?

Yours is the attitude of someone who must conquer or have nothing. If they are smarted once, they will always lie low and be the kind of meek one for whom no person could have sympathy. My friend, I am sorry for you; yours is the way of someone who will never know how to live.

Do not think that logicians have buried their tools and wept. No, they have kept working all this time, no matter how near ambiguity lay. In fact, clarity—the design of logic—and precision—the aim of logicians—gain their power from its nearness. You would see that they are connected if you would only LOOK. Were everything so precise, so clear to us there might have been little left for the logician to do. In the end, that would have been a much more disappointing world.

Fortunately, that world is not ours. Have heart, a possible world may present itself in time. In actuality, logicians have expanded the logical world to create logical worlds, and this they did despite surrounding fog, which is an accomplishment for any theoretical undertaking. From the great and once lone classical logic, they have multiplied not just how many logics there are but also how many notions of logic we may have. These two are bound together anyway, but sometimes we forget that what there is to see affects what we may understand.

We are ready to meet a few of the many that wait for us ahead. We will see their differences and their commons and respect all together. We will see how their lines create new understanding from destroying old assumptions, and how a view can shape our logical world. Finally, may we forswear any remaining instinct that suggests insurmountable opposition and singular domination. For that logic is an imperial logic.¹⁷

Logic’s views

Sometime ago, I presented to you a theory of following logically. I am sure you have since discarded it so I shall give it to you again:

“We say that the sentence $X$ follows logically from the sentences of the class $\mathfrak{R}$ if and only if every model of the class $\mathfrak{R}$ is at the same time a model of the sentence $X$.”¹⁸

It is intimately related to a much wider theory, who sometimes inspires the view that “the relation of a sentence to its models is the cornerstone of all semantics.”¹⁹ This is model theory.

Model Theory is not so esoteric as you might think: it is the study of what you have done heretofore. All this time, and at every turn of the word, you have watched me and INTERPRETED what you saw. It is exasperating that I cannot control how you see so that you would need not interpret. And it is maddening that for what I can control, what there is to see, I may control it only negligibly.

After all, would you have elected to remain by me were it the case that all these words were so fundamentally unreadable & uninterpretable?

Think of sense—significance.

PH 303 by Clyfford Still. Clyfford Still Museum.
If you similarly understand ‘sense,’ you would agree that everything contained from ‘Think’ to now has its sense.

Using this sense of sense:
Imagine that every word of mine was much more SENSELESS.
Something more SENSELESS than a falling leaf in Spring or a drop falling into sand. That is, something so profoundly SENSELESS that I cannot even talk about it or tell you its name. It should be clear that I do not mean ‘something yet to be interpreted’ like a syntactical sign. I have not the words to describe something that is nothing to me. Of course, I am failing now too. I may as well be blathering, or aren’t I already? but my failure is necessary.

Were it so, there would be nothing there for you to interpret, and you would not have grasped that there was anything there to interpret in the first place. So do not pretend that you would have kept by me. You would only look foolish.

Sometimes you will interpret me as I intend. Other times, your interpretation will be far away. Yet no matter what it is, I will either be interpreted or reinterpreted. I, anyway, cannot prevent it.

To be clear, model theory is the mathematical study of the interpretations of any language. Here, its interest for us is that in the historical development of logic, it gave one SHAPE to our understanding of the logical. ²⁰ There are, in fact, two ways that we have more-or-less SHAPED our conception of the logical since the time of Boole and Frege.²¹

ONE is logic as calculus, which is to understand logic in a model-theoretic fashion.

TWO is logic as language, which is nothing less than a universal medium.²²

For your eyes, I have placed two lenses so that you may understand how perception shapes a view.

Perception gives you your place in a room. But no matter how the matter really is, room or no room, your perception will shape how you move in what you perceive. The perceived world is driven by your perception, your perception determines how you move, how you move determines how your surroundings move in response and these together create your view. And then the process may proceed in reverse as your view alters what you see and how you move. This, in fact, it must do, for how could you have initially perceived a room without a view? And so the process goes, moving clockwise and counterclockwise.

These metaphors may not be true in our world, but they are true in the logical world.

Le Jour (Day) by Odilon Redon.
The Metropolitan Museum of Art.

A way of viewing shapes, and eventually becomes shaped by, activity and understanding: that much is clearly seen in those logicians who had divided themselves between ONE and TWO.²³ But what do we see when we see with logic? Do we see our rock-collided, ocean-covered, and core-solidified physical world?

Or rather do we see something more like,

Whether logic helps us more clearly see the world or abstractions, and there is no reason to think that it could not be both all logics share the characteristic that they are principled, irrespective of whether those principles align with any liquid intuition. Moreover, we can make symbol-pieces and make them so that they are forced to move in accordance with those principles. These two things, principles and symbol-pieces, when combined with any domain of play, whether or not it is boundless determine every possible configuration. Here, I mean not just every possible formula or sentence. I mean as well every possible valid formula AND every possible valid sequence of moves that follow one after the other in succession. This is otherwise known as an argument or derivation.

Thus to reconstruct what we see with logic and how so, we might walk in reverse and first ask what do we do?

We begin with principles.

Classical logic has its principles, and some of the most notable are

1. LAW OF EXCLUDED MIDDLE
2. BIVALENCE
3. PRINCIPLE OF NON-CONTRADICTION
4. PRINCIPLE OF EXTENSIONALITY.

All of its principles, along with the conventional symbol-pieces that accord to them, make valid

1. $\neg A \to (A \to B)$
2. $\bot \to A$
3. $A \lor \neg A$
4. $\neg(A \land \neg A)$

among others.

Despite their origin in choice and inheritance, these principles were lauded as the ultimate principles of thought and language sometimes even the world.²⁴However, if language—or thought—is the ultimate activity, then there can be no other logical game that is, no other activity that goes against its principles. Today, we can see this for what it is: an old assumption. to uncover an assumption is to attempt to eliminate it. The world has changed many times over since then, and I include here the logical world. We made, and continue to make, new logics that do not always agree with classical principles.

The non-classical logics are, in fact, not so differently constructed to bar their addition to the concept of logic.

IT IS JUST THAT SOME USE CERTAIN PRINCIPLES WHERE OTHERS DO NOT. Our choice of principles, of course, affects our choice of symbol-pieces that must move in accordance to them. These together will then determine our possible configurations and, ultimately, who can follow whom in sequence. Here again appears consequence, another testament to its importance. Owing to this, you may think of each logic as having, in some measure, their own sense of following. IN OTHER WORDS, THEY ARE MADE IN THE SAME WAY ALBEIT OF DIFFERENT MATERIAL: THIS IS THE EXTENT OF THEIR DIFFERENCE.

Shall we see for ourselves what these games look like?

Here is Łukasiewicz logic, and there is something I cannot yet make out. But it is too far, and I will not seek to disturb it.

Immediately before us:

1. LAW OF EXCLUDED MIDDLE
2. BIVALENCE
3. PRINCIPLE OF NON-CONTRADICTION
4. PRINCIPLE OF EXTENSIONALITY.

Łukasiewicz logic, $Ł_3$, is a many-valued logic, and being a many-valued logic, it first and foremost ignores BIVALENCE.

The idea that every meaningful sentence must be either true or false as a matter of general law is one that Łukasiewicz thought to be an “unshakeable dogma” of Western thought.²⁵ Though, you may also see that the idea of a general logical law is itself dogmatic; there may only be principles for each logical world. ²⁶

$Ł_3$ similarly ignores

THE LAW OF EXCLUDED MIDDLE:

Either it is the case or it is not the case holds for every formula. It is written formally as $A \lor \neg A$. this is  not the same as bivalence.

THE PRINCIPLE OF NON-CONTRADICTION:

It is not the case that it is and is not the case holds for every formula. It is written formally as $\neg(A \land \neg A)$.

Propositional constants and connectives²⁷:

1. $\bot$ for falsity &
2. $\neg$ for negation &
3. $\land$ for conjunction &
4. $\lor$ for disjunction &
5. $\to$ for conditional.

Łukasiewicz logic matrix²⁸:

1. The standard propositional language $\mathcal{L}_0$, including in particular formulas, with $\neg, \land, \lor, \to$.

2. The set of truth values $V = \{\mathbb{T, U, F}\}$.

3. $\mathbb{T}$ is the only designated value, i. e., $V^{+} = \{\mathbb{T}\}$.

4. Truth functions are given by the following tables:

    

   

    

Our domain of play will define for us how to interpret the notions of formula and matrix, and it will furnish the meanings of valuation and satisfaction for $Ł_3$.²⁹

From these preceding sections alone, it will not be completely obvious how $Ł_3$ moves. That is because logic is in part AN ACTIVITY. You will glimpse how it moves only after you have watched someone use the symbol-pieces within $Ł_3$’s domain of play. Once in movement, consequence will certainly make itself visible as you discover which consequence relations obtain in $Ł_3$, and by this process, you will gain a practical understanding of why consequence is the logical world’s core.

Watch my words carefully now friend. Do not let them glide away: I can point and prime so that you are in the best position to see. Nevertheless, regardless of which words I beg, I will never have the means to make you understand how a logic moves. It would be like describing a color: to really see it, you must try to see it for yourself.

It is too late to reject the possibility of a true contradiction only because I have used it against you.³⁰ I will reject whatever case you make since I have long used contradictions that did not explode into triviality³¹ : I have said that we may create as we destroy, and I have talked of something that is nothing. But in all that time, you did not protest.

I must reject your claim again. I know not whether possibilities are real in the way that we are real.

But my response is this:

And if it is not nothing, COULD  YOU STILL DENY POSSIBILITY?

You see, then, we often talk in terms of possibility. After all, we have considered many possibilities on our way here. Though I know you knew this: you simply wished to protest now before never.

I am not chiding you: yours was a good challenge, which I have not yet honestly met. It seems that if anything, possibility is at least real in concept. It is something that is one part of our view of the logical world since, as we saw in earlier steps, our view suggests what is possible in it.

Yet, as much as that possibility and impossibility readily imprint themselves on us, these are concepts that cannot be well-expressed using only classical principles.

THE LOGIC OF POSSIBILITY, A SPECIES OF ‘MODAL LOGIC’, IS AN INTENSIONAL LOGIC NOT AN EXTENSIONAL LOGIC. 

Every intensional logic involves concepts that intuitively cannot be reconciled with THE PRINCIPLE OF EXTENSIONALITY. This principle, and it is one on which classical logic depends, has that you may substitute an object for its identical in any sentence. ³²

It cannot be so with possibility and impossibility. Think of me, for instance:

There can be no guarantee that both sentences share the same fate in truth or falsity were S—H—E and I the same person. For even if S—H—E and I were the same, it may happen that you did not know it. Thus, you could know that I wrote it without knowing that S—H—E , whoever she is, wrote it too.

Having recognized such a problem, we know that a logic of possibility must reject the principle, and so again we must look for the appropriate symbol-pieces and domain of play.

In our last steps taken together, we had tried to approach logical worlds, the motive force of our exploration. We saw their principles, noted their constructions, and enjoyed their passing views. We came nearest to logic as activity when we witnessed the parts constitutive of $Ł_3$. Though there, we still did not see fully what we do.

Turn left to me.

Straight ahead is the short way.

A proof is simply a complete demonstration that some sentence—some formulaic configuration—follows from others or from nothing. To follow from others means we prove from a beginning configuration, which are our assumptions. To follow from nothing means we prove using the symbolic-pieces and domain of play alone; we would begin with no configuration.

Do we understand now what we see in logic? At least for myself, it is not yet clear.

What if we instead had this?

Would that refutation be true?

I myself could not know how to respond, but we may attempt it together.

We would first need to ascertain the proper ‘material’ of a proof. Is a logical proof a complete demonstration in abstraction? Or is it instead a complete demonstration in the physical world?

If we mark a logical proof by its physical existence, the refutation would be false, which is desirable. However, the two preceding proofs would be different proofs. It may well be that THIS IS WHAT SHOULD BE THE CASE given other facts and aims. Yet we know that this DOES NOT RESPECT OUR PRESENT INTUITIONS, LIQUID AS THEY ARE.

This suggests that a proof is made of abstraction, which suggests that THE LOGICAL WORLD WE SEE IS ALSO AN ABSTRACTION.

Even though it would make the refutation true, this view of logic is not so unreasonable: we have, in some sense, constructed a language whose parts are made of logical ‘forms’ that are structured and related immaterially.³³

I have long suggested that form cannot be so easily pulled from content. Form, in fact, clarifies content just as it may distort: I once said, “there is not one thing to find.” Did you consider whether I meant,

These are clearly two different meanings that form alone has brought to surface. The difference is not negligible: it would have changed your interpretation and understanding, which are changes that could have affected a part if not the whole.

If you believe this is still so little and so trite, I have a demonstration for you that is larger yet. Take everything here as your challenge: Copy my words, set them plainly elsewhere, remove spaces that divide paragraphs or even words! and black lines that obscure, eliminate dots, and eliminate precise positions. Does it still say as much? Order is a part of form so put paragraphs forget words into disarray and reorder the sequence of following in the proof. Does it still say as much? Should I still grant you punctuation and images? It remains disastrous even if I should grant them. But if I did not, DOES IT STILL SAY AS MUCH?

The logical world would suffer the same
if you dealt it with the same violence.
the possibility of its meaning for humans
depends much on its perceivable form.
Hence it cannot be only abstraction.

In walking on this road,
through an expanse of logical worlds,
we together saw that
between two,
a choice must always be made
is an old assumption.

O! How there has become here, even in fog and uncertainty! And how here reveals yet another there as a reminder of the wideness of our world and worlds. O my dear companion, you who remained, let me confess at last:

Home References About A Spare Page